Journal of Mathematical Sciences: Advances and Applications[1] R. Andreani, A. Friedlander and S. A. Santos, On the resolution of
the generalized nonlinear complementarity problem, SIAM J. Optim. 12
(2001), 303-321.
[2] C. Geiger and C. Kanzow, On the resolution of monotone
complementarity problem, Comput. Optim. Appl. 5 (1996), 155-173.
[3] M. Kojima, N. Megiddo and A. Yoshise, An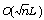 
iteration potential reduction algorithm for
linear complementarity problems, Math. Prog. 50 (1991), 331-342.
[4] K. Tanabe, Centered Newton Method for Mathematical Programming
[C], M. Iri and K. Yajima, eds., System Modeling and Optimization:
Procedings of the 13th IFIP Conference, Lecture Notes in Control and
Information Systems, (1988), pp. 197-206.
[5] M. J. Todd and Y. Ye, A centered projective algorithm for linear
programming, Math. Oper. Res. 15 (1990), 508-529.
[6] Y. J. Wang, F. M. Ma and J. Z. Zhang, A nonsmooth L-M method for
solving the generalized nonlinear complementarity problem over a
polyhedral cone, Appl. Math. Optim. 52 (2005), 73-92.
[7] A. Yoshise, Complementarity Problems, Interior Point Methods of
Mathematical Programming, T. Terlaky, eds., 1996.
[8] X. Z. Zhang, F. M. Ma and Y. J. Wang, A Newton-type algorithm for
generalized linear complementarity problem over a polyhedral cone,
Appl. Math. Comput. 169 (2005), 388-401.
