Journal of Mathematical Sciences: Advances and Applications[1] R. Badora, On Hyers-Ulam stability of Wilson’s functional
equation, Aequationes Math. 60 (2000), 211-218.
[2] J. A. Baker, The stability of the cosine equation, Proc. Amer.
Math. Soc. 80 (1980), 411-416.
[3] J. A. Baker, J. Lawrence and F. Zorzitto, The stability of the
equation 
Proc. Amer. Math. Soc. 74 (1979),
242-246.
[4] B. Bouikhalene, On Hyers-Ulam stability of generalised
Wilson’s equations, J. Inequal. in Pure & Appl. Math. 5(4)
(2004), Article 100.
[5] I. Corovei, The functional equation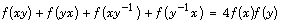 
for nilpotent groups, Buletinul¸
Ştiinţific al Institutului Politehnic Cluj-Napoca 20 (1977),
25-28.
[6] I. Corovei, The functional equation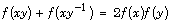 
for nilpotent groups, Mathematica (Cluj)
22(45) (1980), 33-41.
[7] T. M. K. Davison, D’Alembert’s functional equation
on topological monoids, Publ. Math. Debrecen 75(1-2) (2009), 41-66.
[8] E. Elqorachi and M. Akkouchi, The superstability of the
generalized d’Alembert functional equation, Georgian Math. J.
10(3) (2003), 503-508.
[9] E. Elqorachi and M. Akkouchi, On Hyers-Ulam stability of Cauchy
and Wilson equations, Georgian Math. J. 11(1) (2004), 69-82.
[10] E. Elqorachi and M. Akkouchi, On generalized d’Alembert
and Wilson functional equations, Aequationes Math. 66(3) (2003),
241-256.
[11] D. H. Hyers, On the stability of the linear functional equation,
Proc. Nat. Acad. Sci. U.S.A. 27 (1941), 222-224.
[12] D. H. Hyers, G. I. Isac and Th. M. Rassias, Stability of
Functional Equations in Several Variables, Progress in Nonlinear
Differential Equations and their Applications, 34, Birkhauser Boston,
Inc., Boston, MA, 1998.
[13] P. Kannappan, The functional equation 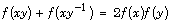for groups, Proc. Amer. Math. Soc. 19
(1968), 69-74.
[14] Th. M. Rassias, On the stability of linear mapping in Banach
spaces, Proc. Amer. Math. Soc. 72 (1978), 297-300.
[15] H. Stetkaer, D’Alembert’s and Wilson’s
functional equations on step 2 nilpotent groups, Aequationes Math.
67(3) (2004), 241-262. 39B32 MR2162231 (2006h:39030).
[16] L. Székelyhidi, On a theorem of Baker, Lawrence and Zorzitto,
Proc. Amer. Math. Soc. 84 (1982), 95-96.
[17] L. Székelyhidi, On a stability theorem, C. R. Math. Rep. Acad.
Sci. Canada 3 (1981), 253-255.
[18] S. M. Ulam, A Collection of Mathematical Problems, Interscience
Publ. New York, 1961; Problems in Modern Mathematics, Wiley, New York,
1964.
[19] D. Zeglami, S. Kabbaj, A. Charifi and A. Roukbi, 
Functional Equations and Hyers-Ulam
Stability Problem in Hypergroups, Functional Equations in Mathematical
Analysis, Springer Optimization and its Applications 52, DOI
10.1007/978-1-4614-0055-4 26.
