Author's: Daniel Abraham Romano
Pages: [121] - [128]
Received Date: December 01, 2008
Submitted by:
This article, within the Bishop’s constructive mathematics, is
a continuation of author’s investigation of anti-orders on set
with apartness. An anti-order 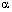 is complete if
is complete if 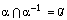 holds. The result of this investigation is
the following: A complete anti-order
holds. The result of this investigation is
the following: A complete anti-order 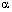 is the intersection of family of all
anti-orders containing
is the intersection of family of all
anti-orders containing 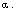
constructive mathematics, set with apartness, anti-order, complete anti-order, quasi-antiorder.