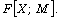Journal of Mathematical Sciences: Advances and ApplicationsAuthor's: Ryan Gipson
Pages: [21] - [45]
Received Date: January 8, 2021
Submitted by:
DOI: http://dx.doi.org/10.18642/jmsaa_7100122170
We consider general factorization properties of particular classes of
integral domains. More specifically, we investigate the bounded
factorization property of the monoid domain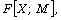 
where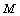 
is an additive submonoid of the nonnegative
rational numbers, a ring of polynomial-like expressions where
exponents come from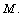 
We accomplish this task by examining sufficient
and necessary factorization properties of the associated monoid
and, in particular, its irreducibles. We divide
our work into two sections: we first consider those bounded
factorization domains with the finite factorization property, and,
finally, we characterize all bounded factorization monoid domains by
precisely determining the necessary factorization properties of its
associated monoid.
atomic domain, bounded factorization domain, monoid domain 
