Volume no :18, Issue no: 1-2, November and December 2012

Journal of Mathematical Sciences: Advances and Applications

UNCONDITIONAL BANACH SPACE IDEAL PROPERTY

Author's: MUSUNDI SAMMY, SHEM AYWA, JAN FOURIE and JOHN WANYONYI MATUYA
Pages: [21] - [28]
Received Date: July 14, 2012
Submitted by:

Abstract

Let 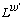denote the assignment which associates with each pair of Banach spaces 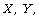the vector space and 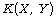be the space of all compact linear operators from X to Y. Let 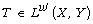and suppose 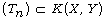converges in the dual weak operator topology 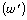of T. Denote by 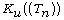the finite number given by



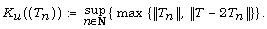
The u-norm on is then given by



It has been shown that is a Banach operator ideal. We find conditions for 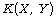to be an unconditional ideal in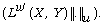

Keywords

ideal projection, separable reflexive space, unconditional compact approximation property (UKAP), u-ideal.