[1] Y. Ait-Sahalia and J. Jacod, Estimating the degree of activity of
jumps in high frequency data, The Annals of Statistics 37(5A) (2009),
2202-2244.
DOI: https://doi.org/10.1214/08-AOS640
[2] Y. Ait-Sahalia and J. Jacod, High-Frequency Financial
Econometrics, Princeton University Press, 2014.
[3] Y. Ait-Sahalia and D. Xiu, Increased correlation among asset
classes: Are volatility or jumps to blame, or both?, Journal of
Econometrics 194(2) (2016), 205-219.
DOI: https://doi.org/10.1016/j.jeconom.2016.05.002
[4] J. Bai, Testing parametric conditional distributions of dynamic
models, The Review of Economics and Statistics 85(3) (2003),
531-549.
DOI: https://doi.org/10.1162/003465303322369704
[5] K. Balcombe and I. Fraser, Do bubbles have an explosive signature
in Markov switching models?, Economic Modelling 66 (2017), 81-100.
DOI: https://doi.org/10.1016/j.econmod.2017.06.001
[6] A. Behme, C. Kluppelberg and K. Mayr, Asymmetric COGARCH
processes, Journal of Applied Probability 51(A) (2014), 161-173.
DOI: https://doi.org/10.1239/jap/1417528473
[7] T. Bollerslev, Generalized autoregressive conditional
heteroskedasticity, Journal of Econometrics 31(3) (1986), 307-327.
DOI: https://doi.org/10.1016/0304-4076(86)90063-1
[8] George E. P. Box and Norman R. Draper, Empirical Model-Building
and Response Surfaces, p. 424, Wiley, 1987.
[9] P. J. Brockwell and R. A. Davis, Time Series: Theory and Methods,
2nd Edition, New York: Springer, 1991.
[10] C. Brooks and A. Katsaris, A three-regime model of speculative
behaviour: Modelling the evolution of the S&P 500 composite index, The
Economic Journal 115(505) (2005), 767-797.
DOI: https://doi.org/10.1111/j.1468-0297.2005.01019.x
[11] V. Cermak, Can Bitcoin Become a Viable Alternative to Fiat
Currencies? An Empirical Analysis of Bitcoin’s Volatility Based
on a GARCH Model, 2017.
DOI: http://dx.doi.org/10.2139/ssrn.2961405
[12] A. Cheung, E. Roca and J. Su, Cryptocurrency bubbles: An
application of the Phillips-Shi-Yu (2013) methodology on Mt. Gox
Bitcoin prices, Applied Economics 47(23) (2015), 2348-2358.
DOI: https://doi.org/10.1080/00036846.2015.1005827
[13] J. Chu, S. Chan, S. Nadarajah and J. Osterrieder, GARCH modelling
of cryptocurrencies, Journal of Risk and Financial Management 10(4)
(2017), Article 17.
DOI: https://doi.org/10.3390/jrfm10040017
[14] CoinMetrics, Cryptocurrency Market Capitalizations, (accessed on
5 November 2017), 2017.
Available online: https://coinmetrics.io/data-downloads/
[15] V. Corradi and N. R. Swanson, Bootstrap conditional distribution
tests in the presence of dynamic misspecification, Journal of
Econometrics 133(2) (2006), 779-806.
DOI: https://doi.org/10.1016/j.jeconom.2005.06.013
[16] G. M. Caporale and T. Zerokh, Modelling Volatility of
Cryptocurrencies Using Markov-Switching Garch Models, CESifo Working
Paper No. 7167 (2018).
[17] J.-C. Duan, The GARCH option pricing model, Mathematical Finance
5(1) (1995), 13-32.
DOI: https://doi.org/10.1111/j.1467-9965.1995.tb00099.x
[18] J.-C. Duan, Augmented GARCH (p, q) process and its
diffusion limit, Journal of Econometrics 79(1) (1997), 97-127.
DOI: https://doi.org/10.1016/S0304-4076(97)00009-2
[19] J.-C. Duan, G. Gauthier and J.-G. Simonato, An analytical
approximation for the GARCH option pricing model, Journal of
Computational Finance 2(4) (1999), 75-116.
DOI: https://doi.org/10.21314/JCF.1999.033
[20] J.-C. Duan, G. Gauthier, C. Sasseville and J.-G. Simonato,
Analytical approximations for the GJR-GARCH and EGARCH option pricing
models, Tech. Rep. G-2004-82, Les Cahiers du GERAD (2004).
[21] R. F. Engle and T. Bollerslev, Modelling the persistence of
conditional variances, Econometric Reviews 5(1) (1986), 1-50.
DOI: https://doi.org/10.1080/07474938608800095
[22] R. F. Engle, Autoregressive conditional heteroscedasticity with
estimates of the variance of United Kingdom inflation, Econometrica
50(4) (1982), 987-1007.
DOI: https://doi.org/10.2307/1912773
[23] R. F. Engle and V. K. Ng, Measuring and testing the impact of
news on volatility, The Journal of Finance 48(5) (1993), 1749-1778.
DOI: https://doi.org/10.1111/j.1540-6261.1993.tb05127.x
[24] V. Fasen, C. Kluppelberg and A. Lindner, Extremal behavior of
stochastic volatility models, In: A. N. Shiryaev, M. R. Grossinho, P.
E. Oliviera and M. Esquivel (Editors), Stochastic Finance, New York:
Springer (2005), 107-155.
[25] C. Francq and J.-M. Zakoian, GARCH Models: Structure, Statistical
Inference and Financial Applications, 2010.
[26] K. Ghoudi and B. Remillard, Comparison of specification tests for
GARCH models, Computational Statistics & Data Analysis 76 (2014),
291-300.
DOI: https://doi.org/10.1016/j.csda.2013.03.009
[27] L. R. Glosten, R. Jagannathan and D. E. Runkle, On the
relationship between the expected value and the volatility of the
nominal excess return on stocks, The Journal of Finance 48(5) (1993),
1779-1801.
DOI: https://doi.org/10.1111/j.1540-6261.1993.tb05128.x
[28] M. Gronwald, The Economics of Bitcoins - Market Characteristics
and Price Jumps, CESifo Working Paper Series No. 5121 (2014).
Available at: https://ssrn.com/abstract=2548999
[29] C. Gourieroux and A. Monfort, Statistics and Econometric Models,
Cambridge University Press, Cambridge, 1995.
[30] C. Gourieroux and A. Monfort, Time Series and Dynamic Models,
Cambridge University Press, Cambridge, 1996.
DOI: https://doi.org/10.1017/CBO9780511628597
[31] E. Jondeau and M. Rockinger, Conditional volatility, skewness and
kurtosis: Existence, persistence, and comovements, Journal of Economic
Dynamics & Control 27(10) (2003), 1699-1737.
DOI: https://doi.org/10.1016/S0165-1889(02)00079-9
[32] S. G. Hall, Z. Psaradakis and M. Sola, Detecting periodically
collapsing bubbles: A Markov-switching unit root test, Journal of
Applied Econometrics 14(2) (1999), 143-154.
DOI:
https://doi.org/10.1002/(SICI)1099-1255(199903/04)14:2<143::AID-JAE500
>3.0.CO;2-X
[33] C. Kluppelberg, A. Lindner and R. Maller, A continuous-time GARCH
process driven by a Levy process: Stationarity and second-order
behaviour, Journal of Applied Probability 41(3) (2004), 601-622.
DOI: https://doi.org/10.1239/jap/1091543413
[34] C. Kluppelberg, A. Lindner and R. Maller, Continuous Time
Volatility Modelling: COGARCH Versus Ornstein-Uhlenbeck Models, In:
Kabanov, Y., Lipster, R. and Stoyanov, J. (Eds.) From Stochastic
Calculus to Mathematical Finance, The Shiryaev Festschrift (2006),
393-419. Berlin: Springer.
DOI: https://doi.org/10.1007/978-3-540-30788-4_21
[35] P. Katsiampa, Volatility estimation for Bitcoin: A comparison of
GARCH models, Economics Letters 158 (2017), 3-6.
DOI: https://doi.org/10.1016/j.econlet.2017.06.023
[36] D. B. Nelson, ARCH models as diffusion approximations, J.
Econometrics 45 (1990), 7-38.
[37] D. B. Nelson, Conditional heteroskedasticity in asset returns: A
new approach, Econometrica 59(2) (1991), 347-370.
DOI: https://doi.org/10.2307/2938260
[38] E. V. Khmaladze, An innovation approach to goodness-of-fit tests
in 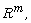 The Annals of Statistics 16(4) (1988),
1503-1516.
The Annals of Statistics 16(4) (1988),
1503-1516.
DOI: https://doi.org/10.1214/aos/1176351051
[39] P. C. B. Phillips, Shu-Ping Shi and J. Yu, Testing for multiple
bubbles: Historical episodes of exuberance and collapse in the S&P
500, International Economic Review 56(4) (2015), 1043-1078.
DOI: https://doi.org/10.1111/iere.12132
[40] Reuters, 2017. https://goo.gl/2n75gk, accessed 5 November
2017.
[41] B. Remillard, Statistical Methods for Financial Engineering,
Chapman and Hall, New York, 2013.
[42] V. E. Troster, Specification and Casualty of Distribution Models,
University Carlos III Madrid, Doctoral Thesis, 2015.
Available via: http://hdl.handle.net/10016/21495
[43] O. Scaillet, A. Treccani and C. Trevisan, High-Frequency Jump
Analysis of the Bitcoin Market, Swiss Finance Institute Research Paper
No. 17-19 (2017).
DOI: http://dx.doi.org/10.2139/ssrn.2982298
[44] S. Shi and V. Arora, An application of models of speculative
behaviour to oil prices, Economics Letters 115(3) (2012), 469-472.
DOI: https://doi.org/10.1016/j.econlet.2011.12.126
[45] O. Vasicek, An equilibrium characterization of the term
structure, Journal of Financial Economics 5(2) (1977), 177-188.
DOI: https://doi.org/10.1016/0304-405X(77)90016-2