Journal of Algebra, Number Theory: Advances and Applications
[1] C. Ding, G. Xiao and W. Shan, The Stability Theory of Stream
Ciphers, Lecture Notes in Computer Science, Springer, Berlin, 1991.
[2] S. W. Golomb, Shift Register Sequences, Aegean Park Press, Laguna
Hills, CA, 1982.
[3] S. W. Golomb and G. Gong, Signal Design for Good Correlation for
Wireless Communication, Cryptography and Radar, Cambridge University
Press, Cambridge, UK, 2005.
[4] T. W. Hungerford, Algebra, New York, Springer-Verlag, 1974.
[5] R. Lidl and H. Niederreiter, Finite Fields, Cambridge University
Press, Cambridge, 1997.
[6] J. Massey, Shift register synthesis and BCH decoding, IEEE
Transactions on Information Theory 15(1) (1969), 122-127.
DOI: https://doi.org/10.1109/TIT.1969.1054260
[7] W. Meidl and H. Niederreiter, The expected value of the joint
linear complexity of periodic multisequences, Journal of Complexity
19(1) (2003), 61-72.
DOI: https://doi.org/10.1016/S0885-064X(02)00009-2
[8] W. Meidl and F. Özbudak, Linear complexity over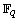 
and over 
for linear recurring sequences, Finite Fields
and their Applications 15(1) (2009), 110-124.
DOI: https://doi.org/10.1016/j.ffa.2008.09.004
[9] W. Meidl and F. Özbudak, Generalized Joint Linear Complexity of
Linear Recurring Multisequences, In: S. W. Golomb et al. (editors),
Sequences and their Applications -SETA 2008, In: Lecture Notes in
Computer Science, Springer (LNCS), Berlin, 5203 (2008), 266-277.
[10] H. Niederreiter, Sequences with Almost Perfect Linear Complexity
Profile, in: D. Chaum and W. L. Price (editors), Advances in
Cryptology-EUROCRYPT 1987, In: LNCS, Springer, Berlin, 304 (1988),
37-51.
[11] Z. H. Gao and F. W. Fu, The minimal polynomial over 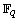of linear recurring sequence over 
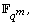Finite Fields and their Applications 15(6)
(2009), 774-784.
DOI: https://doi.org/10.1016/j.ffa.2009.07.002
[12] Z. H. Gao and F. W. Fu, The minimal polynomial of a sequence
obtained from the componentwise linear transformation of a linear
recurring sequence, Theoretical Computer Science 411(44-46) (2010),
3883-3893.
DOI: https://doi.org/10.1016/j.tcs.2010.07.014
[13] R. A. Rueppel, Analysis and Design of Stream Ciphers, New York,
Springer-Verlag, 1986.
[14] L. P. Wang, The Vector Key Equation and Multisequence Shift
Register Synthesis, In: M. Fossorier et al. (editors), Applied
Algebra, Algebraic Algorithms and Error-Correcting Codes, Lecture
Notes in Computer Science, Springer, Berlin, 3857 (2006), 68-75.
[15] Z. X. Wan, Lectures on Finite Fields and Galois Rings, World
Scientific Publish. Co., Singapore, 2003.
