References

Journal of Algebra, Number Theory: Advances and Applications

OF REGULAR SEMIGROUPS WITH INVERSE TRANSVERSALS

[1] B. P. Alimpié and D. N. Krgović, Some congruences on regular semigroups, Lecture Notes in Math. 1320 (1988), 1-10.

[2] Y. Y. Feng and L. M. Wang, Congruences on regular semigroups associated with Green’s relations, Submitted.

[3] Y. Y. Feng and L. M. Wang, The 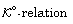on the congruence lattice of a regular semigroup with an inverse transversal, Acta Mathematica Sinica, English Series 28(5) (2012), 1061-1074.
DOI: https://doi.org/10.1007/s10114-011-9112-0

[4] J. M. Howie, Fundamentals of Semigroup Theory, Clarendon Press, London, 1995.

[5] Y. F. Luo, The VT-operator semigroup for two kinds of regular semigroups, Semigroup Forum 59(3) (1999), 374-388.
DOI: https://doi.org/10.1007/PL00022747

[6] Y. F. Luo and L. M. Wang, Semigroups of operators associated with the kernel and trace of congruences on E-unitary completely regular semigroups, Comm. Algebra 29(1) (2001), 99-108.
DOI: https://doi.org/10.1081/AGB-100000787

[7] F. Pastijn and M. Petrich, Congruences on regular semigroups, Trans. Amer. Math. Soc. 295(2) (1986), 607-633.
DOI: https://doi.org/10.1090/S0002-9947-1986-0833699-3

[8] F. Pastijn and M. Petrich, The congruence lattice of a regular semigroup, J. Pure and Appl. Algebra 53(1-2) (1988), 93-123.
DOI: https://doi.org/10.1016/0022-4049(88)90015-1

[9] F. Pastijn and P. G. Trotter, Lattices of completely regular semigroup varieties, Pacific J. Math. 119(1) (1985), 191-214.
DOI: https://doi.org/10.2140/pjm.1985.119.191

[10] M. Petrich, Inverse Semigroups, Wiley, New York, 1984.

[11] M. Petrich, The semigroup generated by certain operators on the congruence lattice of a Clifford semigroup, Semigroup Forum 45(1) (1992), 332-341.
DOI: https://doi.org/10.1007/BF03025774

[12] M. Petrich, Congruence networks for completely simple semigroups, J. Austral. Math. Soc. 56(2) (1994), 243-266.
DOI: https://doi.org/10.1017/S1446788700034868

[13] M. Petrich and N. R. Reilly, A network of congruences on an inverse semigroup, Trans. Amer. Math. Soc. 270(1) (1982), 309-325.
DOI: https://doi.org/10.1090/S0002-9947-1982-0642343-6

[14] T. Saito, Construction of regular semigroups with inverse transversals, Proc. Edinburgh Math. Soc. 32(1) (1989), 41-51.
DOI: https://doi.org/10.1017/S0013091500006891

[15] X. L. Tang, Regular semigroups with inverse transversals, Semigroup Forum 55(1) (1997), 24-32.
DOI: https://doi.org/10.1007/PL00005909

[16] X. L. Tang and L.-M. Wang, Congruences on regular semigroups with inverse transversals, Comm. Algebra 23(11) (1995), 4157-4171.
DOI: https://doi.org/10.1080/00927879508825455

[17] L.-M. Wang, TK-operator semigroups for cryptogroups, Semigroup Forum 60(3) (2000), 368-384.
DOI: https://doi.org/10.1007/s002339910030

[18] L.-M. Wang, Trace-kernel-operator semigroups of bisimple 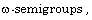Semigroup Forum 60(3) (2000), 424-435.
DOI: https://doi.org/10.1007/s002339910034

[19] L.-M. Wang, On congruence lattices of regular semigroups with Q-inverse transversals, Semigroup Forum 50(1) (1995), 141-160.
DOI: https://doi.org/10.1007/BF02573513

[20] L.-M. Wang and X. L. Tang, Congruence lattices of regular semigroups with inverse transversals, Comm. Algebra 26(4) (1998), 1243-1255.
DOI: https://doi.org/10.1080/00927879808826196