Journal of Statistics: Advances in Theory and ApplicationsAuthor's: Nitis Mukhopadhyay and Bhargab Chattopadhyay
Pages: [93] - [130]
Received Date: April 20, 2011
Submitted by:
We consider unbiased estimation of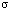 
in a 
population. Traditional unbiased stimators
consist of appropriate multiples of both the sample standard deviation
S, that is,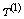 
and Gini’s mean difference (GMD),
that is,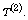 
Both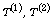 
depend upon U-statistics associated
with symmetric kernels of degree two. In this paper, we develop a new
approach of constructing higher-order unbiased U-statistics
and 
for 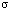based upon symmetric kernels with degree
three, four, and four, respectively. From this investigation, we find
that the new unbiased estimators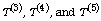 
for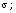 
(i) go practically head-to-head with the
existing estimators 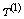and 
(ii) 
beats and (iii)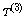 
very nearly beats whether n is small or moderately
large. In other words, it is our belief that this new approach appears
very promising.
efficiency, exact variances, Gini’s mean difference, kernel’s degree higher than two, large-sample variances, population standard deviation, sample standard deviation, symmetric kernel, U-statistics, unbiased estimators.
