Journal of Statistics: Advances in Theory and ApplicationsAuthor's: Ilaria L. Amerise
Pages: [23] - [51]
Received Date: February 6, 2019
Submitted by:
DOI: http://dx.doi.org/10.18642/jsata_7100122031
A novel non-parametric test of rank-order association, denoted as
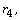has been recently introduced to address the
problem of finding a robust coefficient that, at the same time, is
highly sensitive to differences between rankings. Coefficient
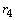has advantages over some best-known
rank-correlations such as Spearman’s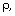 
Kendall’s 
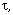and Gini’s 
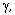in respect of the smoothness of the sampling
distribution and fineness of the resolution.
The properties of 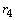have been established for cases in which
rankings do not contain ties. It is the purpose of the present paper
to deal with cases in which ties are present. Several treatments of
ties need to be considered to find the most appropriate one. By using
real and simulated data sets, we find that the Gideon-Hollister method
gives the best procedure to establish how strongly or weakly two
variables are associated when at least one of the rankings contains
ties.
ordinal data, robust rank correlation, independence testing, non-parametric statistics.
