Journal of Algebra, Number Theory: Advances and ApplicationsAuthor's: Y. Talebi, T. Amouzegar and A. Mahmoudi
Pages: [33] - [47]
Received Date: January 20, 2014
Submitted by:
A module M is called closed weak generalized supplemented if
for every closed submodule N of M, there exists a
submodule K of M such that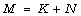 
and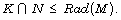 
We prove that for a distributive module
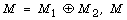is closed weak generalized supplemented, if
and only if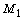 
and 
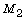are closed weak generalized supplemented.
We characterize nonsingular V-rings in which all nonsingular
modules are closed weak generalized supplemented.
closed submodule, closed weak generalized supplemented module, singular module.
